1. Definition
A guarantee is the assumption of a commitment to pay or perform a contract by the guarantor to a secured entity if the obligor or debtor fails to comply with its contractual commitments.
Likewise, it reduces the credit or contract performance risk of the obligor/debtor and creates contingent liabilities for the guarantor. Concerning the assumption of risks, the guarantor demands compensation from the obligor/debtor as “guarantee fees.”
2. Types of Financial Guarantees Between Related Parties
The creation of a guarantee between entities of the same economic group would be considered as a transaction that would be within the scope of application of the transfer pricing regulations and, in this way, the conditions agreed should be similar to those agreed between independent third parties to comply with the arm’s length principle.
Concerning these operations, it is possible to identify the following models:
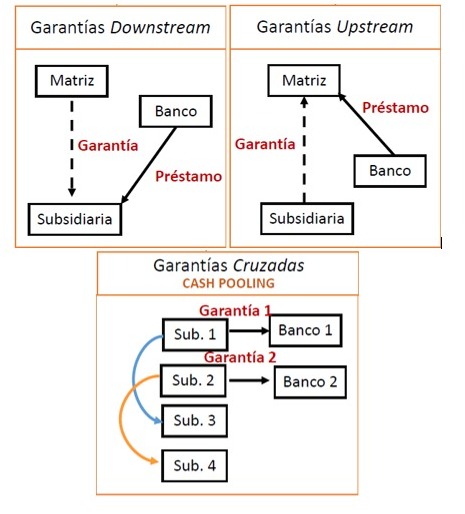
3. Determining Market Value
In order to determine the market value of collateral transactions, the conventional methodologies used in the field of transfer pricing would be applicable. In our experience, when applying these methodologies, we must first identify the existence of internal comparables and, only in the event of their unavailability, resort to external comparables such as specialized databases or market references provided by public bodies, among others.
In addition, the OECD guidelines describe several approaches to determining market values for circumstances in which the guarantee payment is considered appropriate. These include:
Uncontrolled Comparable Price (NCP) Method:
It could be used when there are external or internal comparables. While it might result in a direct comparison, the likelihood of finding comparables is low.
Conversely, there are financial instruments that can be used as proxies to determine the price of these transactions, for example, letters of credit, bank guarantees, surety bonds (similar to guarantee rates), guarantee contracts, and guarantee rates of faithful performance in the local market.
Yield Approach
This approach quantifies the profit the secured party receives from the guarantee in terms of lower interest rates. The method calculates the spread between the interest rate the borrower would pay without the collateral and the interest rate payable with the collateral.
First, determine the interest rate the borrower would have had to pay on his/her own merits, considering the impact of implicit support due to his/her membership in the economic group.
Then, determine the interest rate payable with the benefit of the explicit guarantee. The spread can be used to quantify the profit made by the borrower as a result of the collateral.
The result of this analysis establishes a maximum collateral rate that the recipient of the collateral will be willing to pay. It should be noted that this approach is often used to price financial guarantees due to its simplicity and transparency.
Cost Approach
This method aims to quantify the additional risk assumed by the guarantor by estimating the expected loss incurred by the guarantor in providing the guarantee (loss in the event of default). Alternatively, the expected cost could be determined based on the capital required to bear the risks assumed by the guarantor.
The most widely used models for market pricing under this approach are based on the premise that financial collateral is equivalent to another financial instrument and sets the price of the alternative, for example, by treating the collateral as a put option or a CDS. In this regard, publicly available CDS spreads data can be used to approximate the default risk associated with the loan and, consequently, the collateral fee.
This approach sets a minimum fee that the guarantor should be willing to accept.
Expected Loss Valuation Approach
It estimates the value of a guarantee based on calculating the likelihood of default and adjusts to account for the expected recovery rate in the event of default. This would then be applied to the guaranteed nominal amount to arrive at the cost of providing the guarantee. Examples: probabilistic methods, Value at Risk.
Capital Support Method
Its application is appropriate when the difference between the risk profiles of the guarantor and the borrower can be resolved by putting more capital on the borrower’s balance sheet.
First, the credit rating of the collateral borrower must be determined, and then the amount of additional notional principal necessary for the borrower to achieve the guarantor’s credit rating.
